Busy Beaver puts another one on the Turing Machine's tape (image from the book The New Turing Omnibus).
The busy beaver problem is a fun theoretical computer science problem. Intuitively, the problem is to find the smallest program that outputs as many data as possible and eventually halts. More formally it goes like this – given an n-state Turing Machine with a two symbol alphabet {0, 1}, what is the maximum number of 1's that the machine can print on an initially blank tape (filled with 0's) before halting?
It turns out that this problem can't be solved. For smaller states states, you can reason about it and come up with estimates, but it can't be solved in general. Theorists call such problems non-computable.
Currently people have managed to solve it for n=1, 2, 3, 4 (for Turing Machines with 1, 2, 3 and 4 states) by reasoning about and running all possible Turing Machines, but for n=5 this task has currently been impossible. While it will most likely be solved for n=5, theorists doubt that it shall ever be computed for n=6.
Let's denote B(n) to be the number of 1s that the busy beaver managed to put on the tape. This function B(n) is called the busy beaver function and it is the solution to the busy beaver problem. The busy beaver function is also interesting – it grows faster than any computable function. It grows like this:
- B(1) = 1
- B(2) = 4
- B(3) = 6
- B(4) = 13
- B(5) = 4098 (the exact result has not yet been found)
- B(6) = 4.6 · 101439 (the exact result shall never be known)
If we were to use one atom for each 1 that the busy beaver puts on the tape, then for n=6, we would fill the whole Universe! That's how fast the busy beaver function is growing.
I decided to play with the busy beaver myself to verify the known results for n < 5. I implemented a Turing Machine in Python, which turned out to be too slow, so I re-implemented it in C++ (source code of both implementations below).
I also wrote a visualization tool in Perl that shows how the Turing Machine's tape changes from start to finish (source code also below).
The tapes of Turing Machines are initially filled with 0's. Their starting state is "a" and halting state is "h". The notation "a0 -> b1l" means "if we are in the state "a" and the current symbol on the tape is "0", then put a "1" in that cell, switch to the state "b", and move to the left "l". This process repeats until the machine ends up in the halting state.
Turing Machine for 1-state busy beaver:
a0 -> h1r
Turing Machine for 2-state busy beaver:
a0 -> b1r a1 -> b1l b0 -> a1l b1 -> h1r
Here is how the changes on the tape look like for the 2-state busy beaver:

Tape changes for 2 state busy beaver.
Turing Machine for 3-state Busy Beaver:
a0 -> b1r a1 -> h1r b0 -> c0r b1 -> b1r c0 -> c1l c1 -> a1l
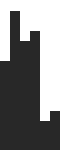
Tape changes for 3 state busy beaver.
Turing Machine for 4-state Busy Beaver:
a0 -> b1r a1 -> b1l b0 -> a1l b1 -> c0l c0 -> h1r c1 -> d1l d0 -> d1r d1 -> a0r
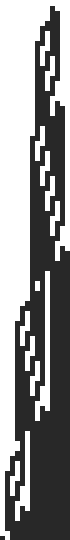
Tape changes for 4 state busy beaver.
Turing Machine for 5-state Busy Beaver:
a0 -> b1l a1 -> a1l b0 -> c1r b1 -> b1r c0 -> a1l c1 -> d1r d0 -> a1l d1 -> e1r e0 -> h1r e1 -> c0r
This image is huge (6146 x 14293 pixels, but only 110KB in size). Click for full size.
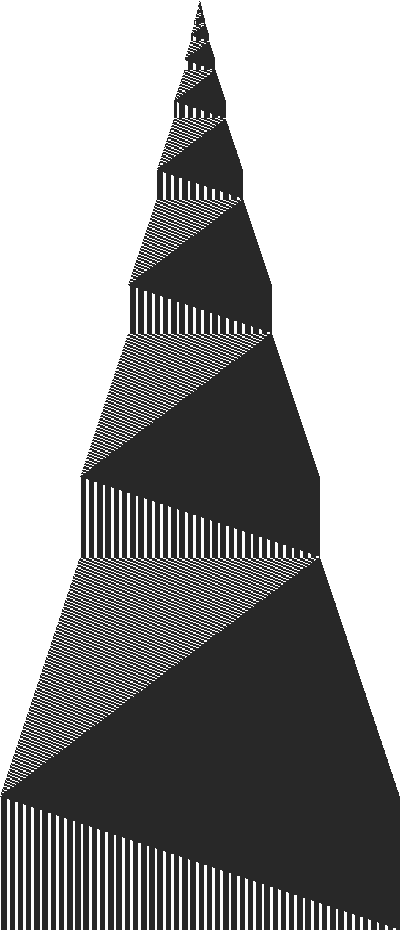
Tape changes for 5 state busy beaver.
Turing Machine for 6 state Busy Beaver:
a0 -> b1r a1 -> e0l b0 -> c1l b1 -> a0r c0 -> d1l c1 -> c0r d0 -> e1l d1 -> f0l e0 -> a1l e1 -> c1l f0 -> e1l f1 -> h1r
Here is my Python program that simulates all these Turing Machines. As I said, it turned out to be too slow and for the 5 state busy beaver it took 5 minutes to generate the currently best known solution.
Download: busy_beaver.py
#!/usr/bin/python
#
# Turing Machine simulator for Busy Beaver problem.
# Version 1.0
#
import sys
class Error(Exception):
pass
class TuringMachine(object):
def __init__(self, program, start, halt, init):
self.program = program
self.start = start
self.halt = halt
self.init = init
self.tape = [self.init]
self.pos = 0
self.state = self.start
self.set_tape_callback(None)
self.tape_changed = 1
self.movez = 0
def run(self):
tape_callback = self.get_tape_callback()
while self.state != self.halt:
if tape_callback:
tape_callback(self.tape, self.tape_changed)
lhs = self.get_lhs()
rhs = self.get_rhs(lhs)
new_state, new_symbol, move = rhs
old_symbol = lhs[1]
self.update_tape(old_symbol, new_symbol)
self.update_state(new_state)
self.move_head(move)
if tape_callback:
tape_callback(self.tape, self.tape_changed)
def set_tape_callback(self, fn):
self.tape_callback = fn
def get_tape_callback(self):
return self.tape_callback
property(get_tape_callback, set_tape_callback)
@property
def moves(self):
return self.movez
def update_tape(self, old_symbol, new_symbol):
if old_symbol != new_symbol:
self.tape[self.pos] = new_symbol
self.tape_changed += 1
else:
self.tape_changed = 0
def update_state(self, state):
self.state = state
def get_lhs(self):
under_cursor = self.tape[self.pos]
lhs = self.state + under_cursor
return lhs
def get_rhs(self, lhs):
if lhs not in self.program:
raise Error('Could not find transition for state "%s".' % lhs)
return self.program[lhs]
def move_head(self, move):
if move == 'l':
self.pos -= 1
elif move == 'r':
self.pos += 1
else:
raise Error('Unknown move "%s". It can only be left or right.' % move)
if self.pos < 0:
self.tape.insert(0, self.init)
self.pos = 0
if self.pos >= len(self.tape):
self.tape.append(self.init)
self.movez += 1
beaver_programs = [
{ },
{'a0': 'h1r' },
{'a0': 'b1r', 'a1': 'b1l',
'b0': 'a1l', 'b1': 'h1r'},
{'a0': 'b1r', 'a1': 'h1r',
'b0': 'c0r', 'b1': 'b1r',
'c0': 'c1l', 'c1': 'a1l'},
{'a0': 'b1r', 'a1': 'b1l',
'b0': 'a1l', 'b1': 'c0l',
'c0': 'h1r', 'c1': 'd1l',
'd0': 'd1r', 'd1': 'a0r'},
{'a0': 'b1l', 'a1': 'a1l',
'b0': 'c1r', 'b1': 'b1r',
'c0': 'a1l', 'c1': 'd1r',
'd0': 'a1l', 'd1': 'e1r',
'e0': 'h1r', 'e1': 'c0r'},
{'a0': 'b1r', 'a1': 'e0l',
'b0': 'c1l', 'b1': 'a0r',
'c0': 'd1l', 'c1': 'c0r',
'd0': 'e1l', 'd1': 'f0l',
'e0': 'a1l', 'e1': 'c1l',
'f0': 'e1l', 'f1': 'h1r'}
]
def busy_beaver(n):
def tape_callback(tape, tape_changed):
if tape_changed:
print ''.join(tape)
program = beaver_programs[n]
print "Running Busy Beaver with %d states." % n
tm = TuringMachine(program, 'a', 'h', '0')
tm.set_tape_callback(tape_callback)
tm.run()
print "Busy beaver finished in %d steps." % tm.moves
def usage():
print "Usage: %s [1|2|3|4|5|6]" % sys.argv[0]
print "Runs Busy Beaver problem for 1 or 2 or 3 or 4 or 5 or 6 states."
sys.exit(1)
if __name__ == "__main__":
if len(sys.argv[1:]) < 1:
usage()
n = int(sys.argv[1])
if n < 1 or n > 6:
print "n must be between 1 and 6 inclusive"
print
usage()
busy_beaver(n)
I rewrote the Turing Machine simulator in C++ and the speedup was huge! Now it took only 14 seconds to execute the same busy beaver 5.
Download: busy_beaver.cpp
/*
** Turing Machine simulator for Busy Beaver problem.
** Version 1.0
*/
#include <cstdlib>
#include <iostream>
#include <utility>
#include <vector>
#include <string>
#include <map>
using namespace std;
typedef vector<char> Tape;
typedef map<string, string> Program;
class TuringMachine {
private:
Tape tape;
Program program;
char start, halt, init, state;
bool tape_changed;
int moves;
int pos;
public:
TuringMachine(Program program, char start, char halt, char init):
tape(1, init), program(program), start(start), halt(halt),
init(init), state(start), moves(0), tape_changed(1), pos(0)
{ }
void run() {
while (state != halt) {
print_tape();
string lhs = get_lhs();
string rhs = get_rhs(lhs);
char new_state = rhs[0];
char new_symbol = rhs[1];
char move = rhs[2];
char old_symbol = lhs[1];
update_tape(old_symbol, new_symbol);
update_state(new_state);
move_head(move);
}
print_tape();
}
int get_moves() {
return moves;
}
private:
inline void print_tape() {
if (tape_changed) {
for (int i=0; i<tape.size(); i++)
cout << tape[i];
cout << endl;
}
}
inline string get_lhs() {
char sp[3] = {0};
sp[0] = state;
sp[1] = tape[pos];
return string(sp);
}
inline string get_rhs(string &lhs) {
return program[lhs];
}
inline void update_tape(char old_symbol, char new_symbol) {
if (old_symbol != new_symbol) {
tape[pos] = new_symbol;
tape_changed++;
}
else {
tape_changed = 0;
}
}
inline void update_state(char new_state) {
state = new_state;
}
inline void move_head(char move) {
if (move == 'l')
pos -= 1;
else if (move == 'r')
pos += 1;
else
throw string("unknown state");
if (pos < 0) {
tape.insert(tape.begin(), init);
pos = 0;
}
if (pos >= tape.size()) {
tape.push_back(init);
}
moves++;
}
};
vector<Program> busy_beavers;
void init_bb6()
{
Program bb6;
bb6.insert(make_pair("a0", "b1r"));
bb6.insert(make_pair("b0", "c1l"));
bb6.insert(make_pair("c0", "d1l"));
bb6.insert(make_pair("d0", "e1l"));
bb6.insert(make_pair("e0", "a1l"));
bb6.insert(make_pair("f0", "e1l"));
bb6.insert(make_pair("a1", "e0l"));
bb6.insert(make_pair("b1", "a0r"));
bb6.insert(make_pair("c1", "c0r"));
bb6.insert(make_pair("d1", "f0l"));
bb6.insert(make_pair("e1", "c1l"));
bb6.insert(make_pair("f1", "h1r"));
busy_beavers.push_back(bb6);
}
void init_bb5()
{
Program bb5;
bb5.insert(make_pair("a0", "b1l"));
bb5.insert(make_pair("b0", "c1r"));
bb5.insert(make_pair("c0", "a1l"));
bb5.insert(make_pair("d0", "a1l"));
bb5.insert(make_pair("e0", "h1r"));
bb5.insert(make_pair("a1", "a1l"));
bb5.insert(make_pair("b1", "b1r"));
bb5.insert(make_pair("c1", "d1r"));
bb5.insert(make_pair("d1", "e1r"));
bb5.insert(make_pair("e1", "c0r"));
busy_beavers.push_back(bb5);
}
void init_bb4()
{
Program bb4;
bb4.insert(make_pair("a0", "b1r"));
bb4.insert(make_pair("b0", "a1l"));
bb4.insert(make_pair("c0", "h1r"));
bb4.insert(make_pair("d0", "d1r"));
bb4.insert(make_pair("a1", "b1l"));
bb4.insert(make_pair("b1", "c0l"));
bb4.insert(make_pair("c1", "d1l"));
bb4.insert(make_pair("d1", "a0r"));
busy_beavers.push_back(bb4);
}
void init_bb3()
{
Program bb3;
bb3.insert(make_pair("a0", "b1r"));
bb3.insert(make_pair("b0", "c0r"));
bb3.insert(make_pair("c0", "c1l"));
bb3.insert(make_pair("a1", "h1r"));
bb3.insert(make_pair("b1", "b1r"));
bb3.insert(make_pair("c1", "a1l"));
busy_beavers.push_back(bb3);
}
void init_bb2()
{
Program bb2;
bb2.insert(make_pair("a0", "b1r"));
bb2.insert(make_pair("b0", "a1l"));
bb2.insert(make_pair("a1", "b1l"));
bb2.insert(make_pair("b1", "h1r"));
busy_beavers.push_back(bb2);
}
void init_bb1()
{
Program bb1;
bb1.insert(make_pair("a0", "h1r"));
busy_beavers.push_back(bb1);
}
void init_busy_beavers()
{
busy_beavers.push_back(Program());
init_bb1();
init_bb2();
init_bb3();
init_bb4();
init_bb5();
init_bb6();
}
void busy_beaver(int n)
{
cout << "Running Busy Beaver with " << n << " states." << endl;
TuringMachine tm(busy_beavers[n], 'a', 'h', '0');
tm.run();
cout << "Busy Beaver finished in " << tm.get_moves() << " steps." << endl;
}
void usage(const char *prog)
{
cout << "Usage: " << prog << " [1|2|3|4|5|6]\n";
cout << "Runs Busy Beaver problem for 1 or 2 or 3 or 4 or 5 or 6 states." << endl;
exit(1);
}
int main(int argc, char **argv)
{
if (argc < 2) {
usage(argv[0]);
}
int n = atoi(argv[1]);
if (n < 1 || n > 6) {
cout << "n must be between 1 and 6 inclusive!\n";
cout << "\n";
usage(argv[0]);
}
init_busy_beavers();
busy_beaver(n);
}
I also wrote a Perl program that uses the GD library to draw the tape changes of a Turing Machine.
Download: draw_turing_machine.perl
#!/usr/bin/perl
#
# Given the output from busy_beaver.py or busy_beaver.cpp programs,
# draw the Turing Machine tape changes (black cell means 1, white 0).
#
use warnings;
use strict;
use GD;
$|++;
my $input_file = shift or die 'Usage: $0 <file with TM state transitions>';
my $cell_size = shift || 4;
my $im_file = "$input_file.png";
sub line_count {
my $count = 0;
open my $fh, '<', shift or die $!;
$count += tr/\n/\n/ while sysread($fh, $_, 2**20);
return $count;
}
sub get_last_line {
my $file = shift;
my $last_line = `tail -1 $file`;
chomp $last_line;
return $last_line;
}
my $nr_lines = line_count $input_file;
my $last_line = get_last_line $input_file;
my $last_width = length($last_line);
my ($width, $height) = ($cell_size*$last_width, $cell_size*$nr_lines);
my $im = GD::Image->new($width, $height);
my $white = $im->colorAllocate(255,255,255);
my $dark = $im->colorAllocate(40, 40, 40);
my ($x, $y) = (0, $height-$cell_size);
print "Starting to draw the image. Total states: $nr_lines.\n";
print "It will be $width x $height pizels in size.\n";
my $prev_line;
my ($pad_left, $pad_right) = (0, 0);
sub pad {
my ($line, $left, $right) = @_;
return '0'x$left . $line . '0'x$right;
}
open my $fh, "-|", "/usr/bin/tac $input_file" or die $!;
while (<$fh>) {
chomp;
print "." if $. % 10 == 0;
print "($.)" if $. % 500 == 0;
$prev_line = $_ unless defined $prev_line;
my $new_line;
if (length $_ != length $prev_line) {
if ($prev_line =~ /0$/) {
$pad_right++;
}
elsif ($prev_line =~ /^0/) {
$pad_left++;
}
else {
die "unexpected data at $. in file $input_file";
}
}
$new_line = pad($_, $pad_left, $pad_right);
$prev_line = $_;
my @cells = split //, $new_line;
for my $cell (@cells) {
$im->filledRectangle($x, $y, $x + $cell_size, $y + $cell_size,
$cell ? $dark : $white);
$x += $cell_size;
}
$y -= $cell_size;
$x = 0;
}
print "\n";
{
open my $fh, ">", $im_file or die $!;
print $fh $im->png;
close $fh;
}
print "Done. Image saved to $im_file.\n";
Feel free to try these programs yourself. Here is how. Run "busy_beaver.py n" with n=1, 2, 3, 4, 5, 6. This will run the n-state busy beaver Turing Machine. The output will be the tape changes. Then use "draw_turing_machine.pl" to visualize the tape changes.
For example:
$ busy_beaver.py 4 > bb4 $ draw_turing_machine.pl bb4
There are also several variations of this problem.
For example, the busiest beaver with 3 and more symbols. See The Busy Beaver Competition for these.
The historical development is also interesting – see The Busy Beaver Historical Survey for more info.
I first learned about the Busy Beaver problem from the book called The New Turing Omnibus. It contains 66 different essays on various computer science subjects such as algorithms, Turing Machines, grammars, computability, randomness, and other fun topics. These essays are written in an accessible style that even high school students can understand them. Each essay is one or two pages long and doesn't take more than 10 minutes to read. I recommend this book. It's one of my favorite books (see all my favorite books).
See you next time!
